ABBA Equation & CA Models
Introduction
The ABBA equation, denoted as [A+B−]/[b−a+], captures the dynamic interactions between components in a system—where “A+” represents an action, “B-” represents a reaction or feedback, and “b-” and “a+” represent the subsequent feedback and adjusted action. This concept can be applied to Cellular Automata (CA) to describe how each cell interacts with its neighbors and evolves over time based on a set of rules.
In a Cellular Automata model, each cell (representing “A+”) has a state that is influenced by its interactions with neighboring cells (the “B-” reaction). These interactions cause changes in the cell’s state, akin to the feedback process described by [A+B−]/[b−a+]. For instance, if a cell’s state (like being “on” or “off”) changes due to the states of its neighbors, this interaction reflects a dynamic feedback loop where one cell’s state affects, and is affected by, adjacent cells.
The relationship between actions (cell states) and reactions (neighboring influences) mirrors the equation’s feedback dynamics, with [b−a+][b−a+] representing how feedback alters the original state, and [A+B−][A+B−] reflecting the initial interaction. This framework helps us understand the evolving behavior of CA systems, showing how local interactions give rise to complex global patterns, such as self-organization and chaos. By applying the [A+B−]/[b−a+] equation, we gain insights into both simulated and natural examples of Cellular Automata, like Lichtenberg figures in electricity, seed dispersion, and water droplets—offering a deeper understanding of complex systems in nature.
To illustrate how the [A+B−]/[b−a+] equation can be applied to Cellular Automata using an example like Lichtenberg figures, let’s break down the process:
Example: Lichtenberg Figures and Cellular Automata
Lichtenberg figures are fractal patterns that emerge when electrical discharges, such as lightning or sparks, interact with surfaces like insulating materials. These figures are created through a process that can be likened to the principles of Cellular Automata (CA), where local interactions between cells evolve into complex, branched structures over time.
Cellular Automata Simulation of Lichtenberg Figures
- Initial State (A+):
- Imagine a grid where each cell represents a potential point for electrical discharge. A specific cell is initialized as “active” (representing an electrical charge), while the others start as “inactive.”
- Interactions with Neighbors (B−):
- The active cell influences its neighboring cells according to specific rules. For instance, in a CA model, an active cell might activate nearby inactive cells if they are within a certain distance or under certain conditions, simulating the spreading of an electrical charge.
- Resulting Feedback (b−):
- As the initially active cell interacts with surrounding cells, some of these cells become active (charged) while others remain inactive. This represents a feedback mechanism where the initial discharge spreads but is also limited by the material’s properties, creating a branching pattern.
- Adjusted State (a+):
- The system adapts as cells transition from active to inactive states after discharging. This adjustment represents how the electric charge dissipates over time, leading to a more stable state once the discharge has spread as far as it can.
- Pattern Formation:
- The process repeats with each iteration of the CA rules, causing the pattern to spread further through the grid. This results in a fractal-like structure that mimics the branching patterns of Lichtenberg figures, where each new branch is influenced by the previous steps of spreading and dissipation ([A+B−]/[b−a+]).
Key Insights:
- The [A+B−] portion reflects how the initial active cell and its interaction with neighbors create a spreading effect, similar to how an electrical discharge branches out.
- The [b−a+] part represents how the system self-regulates and transitions into a new state as cells deactivate after contributing to the spread, just as Lichtenberg figures stabilize after the discharge has completed.
- This CA model helps us understand how complex, fractal-like patterns can emerge from simple, local interactions, similar to how Lichtenberg figures form through the branching of electrical discharges in nature.
By simulating these interactions in a Cellular Automata framework, we can visualize how the dynamic processes of action, reaction, and feedback lead to complex patterns, echoing natural phenomena like Lichtenberg figures. This approach provides a way to study the underlying principles that govern the formation of intricate structures through local rules and emergent behavior.
The ABBA equation [A+B−]/[b−a+][can be related to several Cellular Automata (CA) models, which show how local actions and interactions result in complex emergent patterns through feedback loops. Here are a few examples that demonstrate this relationship:
1. Game of Life
- Model Overview: The Game of Life, created by John Conway, is one of the most famous Cellular Automata models. It consists of a grid of cells that can be either “alive” or “dead” and evolve over time based on simple rules about the state of their neighboring cells.
- Relation to ABBA Equation:
- A+: A cell is in an “alive” state, representing the initial action or state.
- B−: The state of neighboring cells influences whether the cell remains alive or dies (e.g., if a cell has fewer than two live neighbors, it dies due to underpopulation, or with three live neighbors, it remains alive).
- b−: The changes in neighboring cells’ states (e.g., cells dying or coming to life) provide feedback, affecting the entire system’s balance.
- a+: The next generation of the grid represents an adjustment of all cell states based on prior interactions.
- Outcome: The evolving patterns in the Game of Life, like gliders or stable structures, emerge through these local rules and feedback loops, similar to how [A+B−]/[b−a+] describes interactions and adjustments.
2. Forest Fire Model
- Model Overview: In the Forest Fire model, cells represent trees that can be in three states: empty, tree, or burning. The spread of fire follows rules based on neighboring states, creating dynamic patterns of growth and burning.
- Relation to ABBA Equation:
- A+: A cell represents a burning tree, starting the interaction.
- B−: Neighboring trees catch fire if they are adjacent to a burning cell, representing the spread of the fire.
- b−: Burned-out trees become empty cells, providing a negative feedback that slows or stops the spread.
- a+: After burning, the forest gradually regrows, transitioning into a state of renewal where empty cells become new trees.
- Outcome: This process illustrates how the initial action (burning) and feedback (burning out and regrowth) create complex patterns like waves of fire or regeneration, matching the [A+B−]/[b−a+]dynamics.
3. Elementary Cellular Automata: Rule 110
- Model Overview: Elementary Cellular Automata (ECA) involve a simple one-dimensional grid where each cell has a binary state (“0” or “1”) and evolves based on the state of its neighbors. Rule 110 is particularly interesting because it can produce both chaotic and stable patterns.
- Relation to ABBA Equation:
- A+: A cell in state “1” (active) initiates the local interaction.
- B−: Neighboring cells’ states (e.g., “0” or “1”) determine how the active cell changes in the next iteration, influencing the evolution of the pattern.
- b−: As cells update their states, the overall pattern changes, introducing feedback into the system’s evolution (e.g., some regions might stabilize while others remain chaotic).
- a+: The new configuration of “0”s and “1”s in the next generation reflects the adjustments made after the feedback.
- Outcome: Rule 110 demonstrates how simple local interactions can lead to complex, unpredictable structures, similar to [A+B−]/[b−a+] where each step feeds back into the system to create new states.
4. Diffusion-Limited Aggregation (DLA)
- Model Overview: In this CA model, particles randomly move and stick together upon contact, forming complex branching patterns. DLA is often used to model phenomena like crystal growth and electrical discharge patterns.
- Relation to ABBA Equation:
- A+: A particle starts moving through the grid, representing the action.
- B−: When a moving particle encounters a stationary particle, it attaches, creating a feedback effect that changes the environment.
- b−: As particles accumulate, they alter the structure of the grid, providing negative feedback that affects where future particles attach.
- a+: The structure evolves as new particles adjust their paths based on the existing aggregate pattern.
- Outcome: The resulting structure is a fractal-like pattern, similar to Lichtenberg figures. The [A+B−]/[b−a+] relationship shows how the initial movement and subsequent interactions create complex forms through feedback.
These examples show how the principles of the ABBA equation apply to different Cellular Automata models, with each model’s behavior emerging through the interaction between actions, feedback, and adjustments. This approach allows us to understand how local interactions in a CA framework can create the intricate structures and behaviors seen in both simulated environments and natural systems.
Game Of Life Dataset
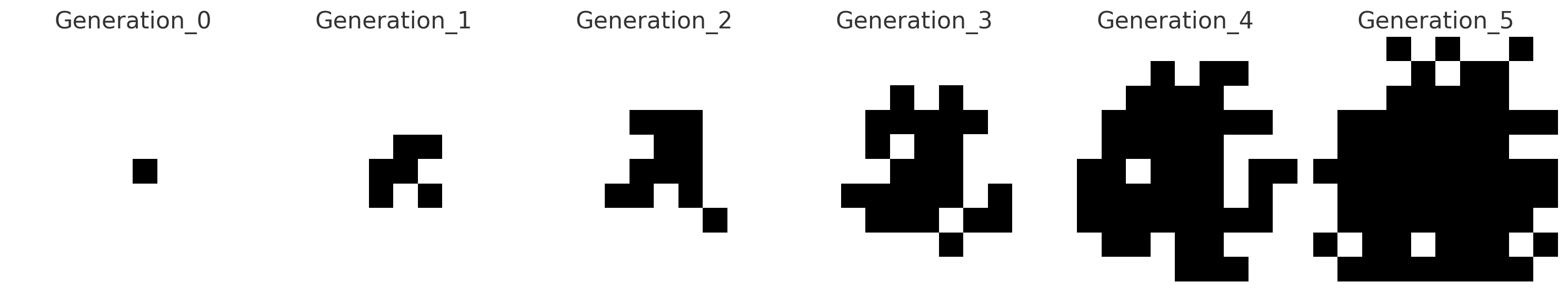
Here is a plot of the Game of Life model over 5 generations on a 5×5 grid. Each panel represents the state of the grid at a specific generation, illustrating how the patterns evolve according to the Game of Life rules.
ABBA Game of Life Dataset
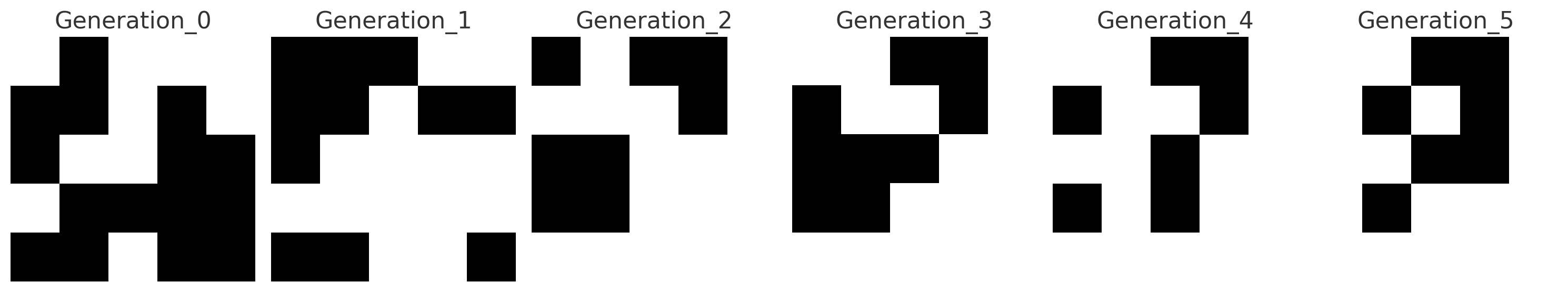
I have generated and plotted a new dataset for the Game of Life model using the ABBA equation logic [A+B−]/[b−a+]. This approach considers the initial cell state (A+), the influence of neighboring cells (B-), the feedback from these interactions (b-), and the final adjusted state (a+). The plot shows how the patterns evolve over five generations with these dynamics.
Lichtenberg ABBA Model Dataset
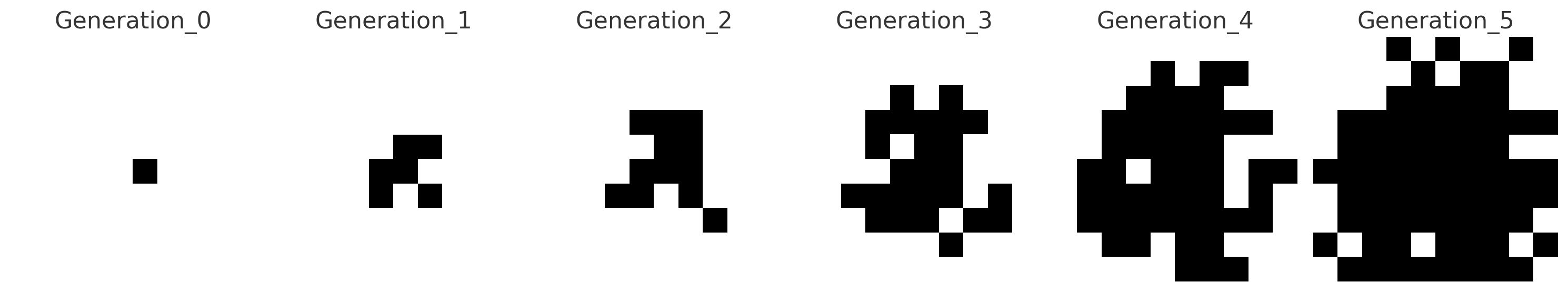
I have generated and plotted a dataset for a Lichtenberg-like model using the ABBA equation logic [A+B−]/[b−a+] over five generations on a 10×10 grid. The model simulates how a central “charge” spreads across the grid, influenced by neighboring cells and introducing some randomness to reflect natural variations seen in Lichtenberg figures. Each step shows the progression of the pattern over generations.
Summary
Modeling using Cellular Automata (CA) and the ABBA equation [A+B−]/[b−a+] can provide valuable insights into analyzing complex structures like the Big Bang Radiation Map or the Cosmic Microwave Background (CMB). CA allows for the simulation of how local interactions between particles or energy states evolve into larger patterns over time, similar to the way fluctuations in the early universe developed into the large-scale structures we observe in the CMB. By applying the ABBA equation, which considers the dynamic relationship between actions, reactions, feedback, and adjustments, researchers can simulate how initial conditions (A+) and subsequent interactions (B−) lead to the self-regulation (b−) and adaptation (a+) seen in cosmic phenomena. This approach can help to better understand the emergence of the CMB’s intricate temperature fluctuations and polarization patterns, providing a framework for exploring how the universe transitioned from a state of high energy density to its current, more stable configuration. The combination of CA and the ABBA equation offers a powerful way to model the evolution of the universe’s structure and the fundamental processes that shaped the cosmic radiation we observe today.